DOC.
1
MANUSCRIPT ON SPECIAL RELATIVITY 49
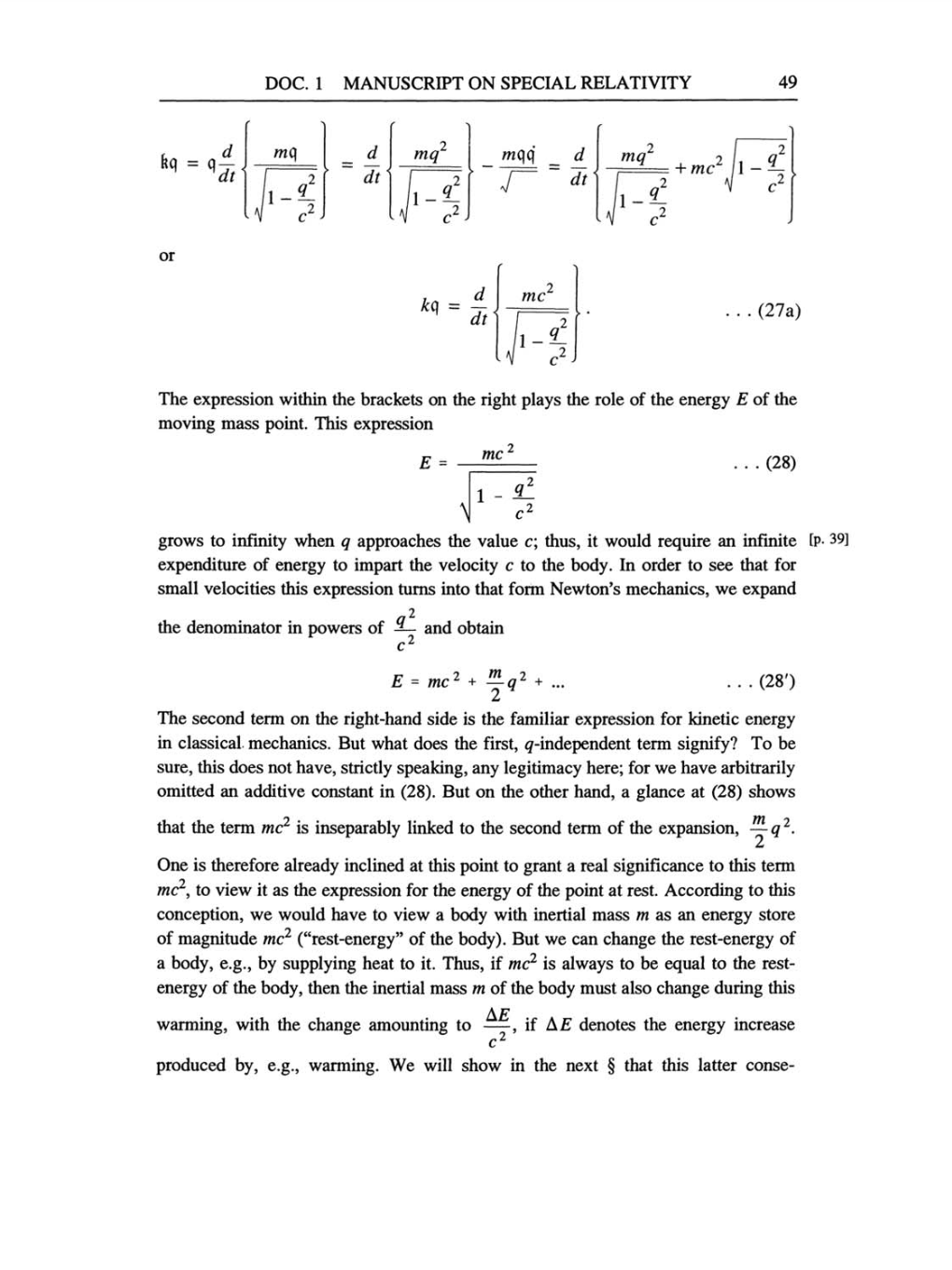
kq
= q
d
dt
1
X
mC|
d
N
mq2 mqq
HI
dt•
IJ-^J
'
J
V
c
J
A
c
d_
dt
mq
+
mc
7
1
or
kq
=
d_
dt1
mc
1
-
7
...
(27a)
The
expression
within the brackets
on
the
right plays
the role of the
energy
E of the
moving
mass
point.
This
expression
E
=
mcl
. . .
(28)
N
i
-q
c2
grows
to
infinity
when
q
approaches
the value
c;
thus,
it
would
require an
infinite
expenditure
of
energy
to
impart
the
velocity
c
to
the
body.
In order
to
see
that for
small velocities this
expression
turns
into that form Newton's
mechanics,
we
expand
the denominator in
powers
of
q2/c2
and obtain
E
=
mc2
+
m/2q2
+
...
. . .
(28')
The second
term
on
the
right-hand
side is
the familiar
expression
for kinetic
energy
in classical mechanics. But what does the
first,
q-independent
term
signify?
To be
sure,
this does
not have,
strictly speaking, any legitimacy
here;
for
we
have
arbitrarily
omitted
an
additive
constant
in
(28).
But
on
the
other
hand,
a glance
at
(28)
shows
that the
term mc2 is
inseparably
linked
to
the second
term
of
the
expansion,
m/2q2.2
One is therefore
already
inclined
at
this
point
to
grant
a
real
significance
to
this
term
mc2,
to
view it
as
the
expression
for the
energy
of the
point
at rest.
According
to
this
conception,
we
would have
to
view
a body
with inertial
mass m as an energy
store
of
magnitude
mc2
("rest-energy"
of the
body).
But
we can
change
the
rest-energy
of
a
body, e.g., by
supplying
heat
to it.
Thus,
if
mc2
is
always
to
be
equal
to
the
rest–
energy
of the
body,
then the inertial
mass m
of
the
body
must
also
change during
this
warming,
with the
change amounting
to
AE/c2,
if AE denotes the
energy
increase
[p. 39]
produced by, e.g., warming.
We will show in
the next
§
that this latter
conse–