DOC. 27 MAXWELL'S
EQUATIONS
133
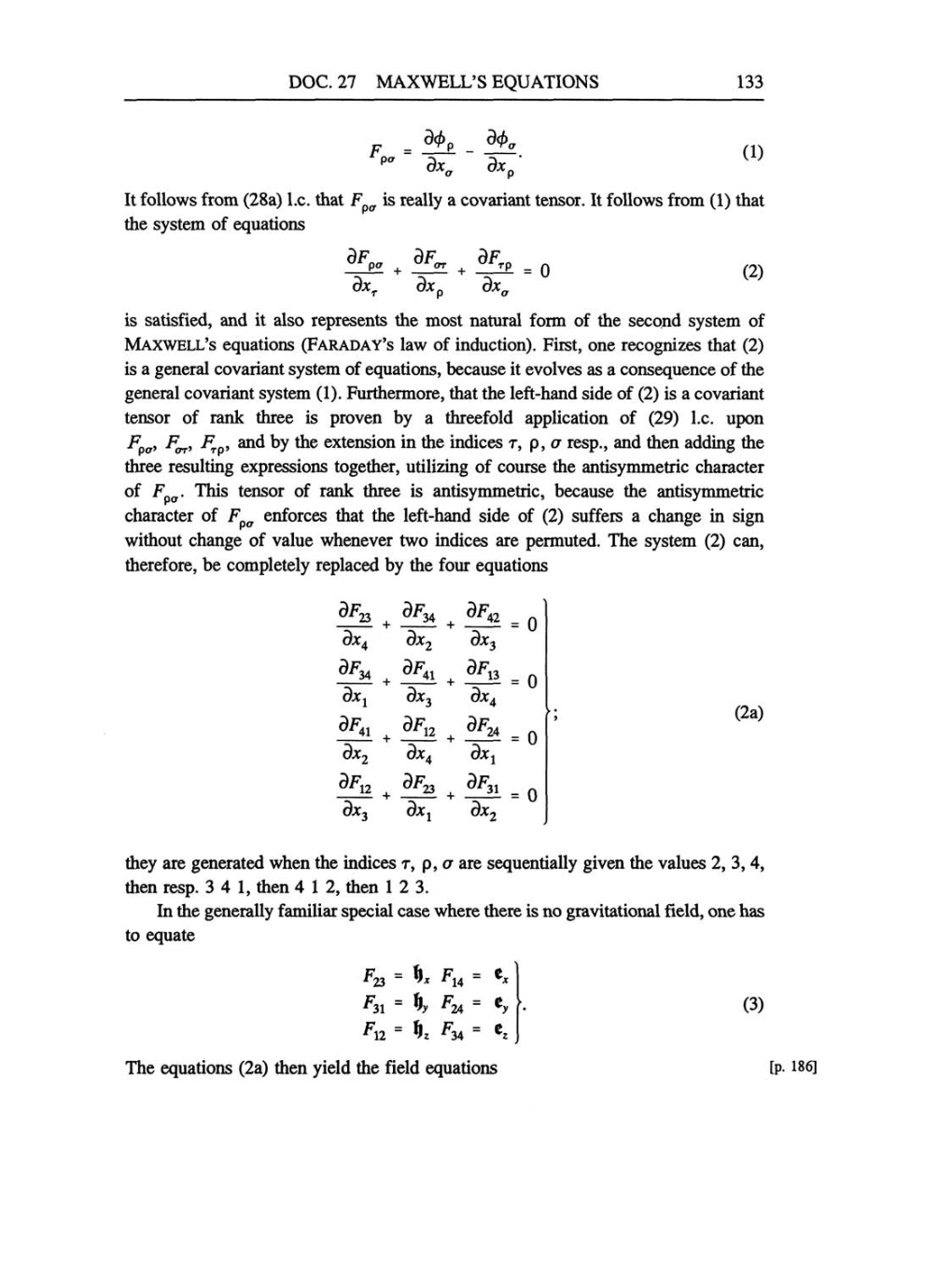
df
F
=
pff dx
p
_
dx
(1)
It follows
from
(28a)
1.c.
that
Fpa
is
really a
covariant tensor. It follows from
(1)
that
the
system
of
equations
^£1
+
dEor
dx_
dF.
TP
-
dx dx
=
0
(2)
is
satisfied,
and it also
represents
the
most
natural form of the second
system
of
Maxwell's
equations
(Faraday's law
of
induction). First,
one recognizes
that
(2)
is
a general
covariant
system
of
equations,
because
it
evolves
as
a
consequence
of
the
general
covariant
system
(1).
Furthermore,
that the left-hand
side
of
(2)
is
a
covariant
tensor
of
rank three is
proven by
a
threefold
application
of
(29)
l.c.
upon
Fpo,
Fm,
Frp,
and
by
the extension in the indices
r,
p,
a
resp.,
and then
adding
the
three
resulting expressions together, utilizing
of
course
the
antisymmetric
character
of
Fpa.
This tensor
of
rank three is
antisymmetric,
because the
antisymmetric
character
of
Fpo
enforces
that
the
left-hand side of
(2)
suffers
a change
in
sign
without
change
of
value whenever two indices
are permuted.
The
system
(2)
can,
therefore,
be
completely replaced by
the four
equations
ÖFjj
+
^34
+
öf42
dx4
dx2 dx3
dFM
+
dp4l
+
dFn
dx1 dx4
dF,
i
+
dFn
+
dx2 dx4
ÖJCj
3F12
+
dF-g
+
dFn
dx3 dx2
=
0
=
0
=
0
=
0
•
9
(2a)
they
are
generated
when the indices
r,
p, a
are sequentially
given
the values
2, 3,
4,
then
resp.
3
4
1,
then 4
1
2,
then
1 2
3.
In the
generally
familiar
special
case
where there
is
no gravitational
field,
one
has
to
equate
^23
"
F3l
=
F\2
=
^
Fu
F24
Fm
=
e
=
c
=
e
(3)
The
equations (2a)
then
yield
the field
equations
[p.
186]