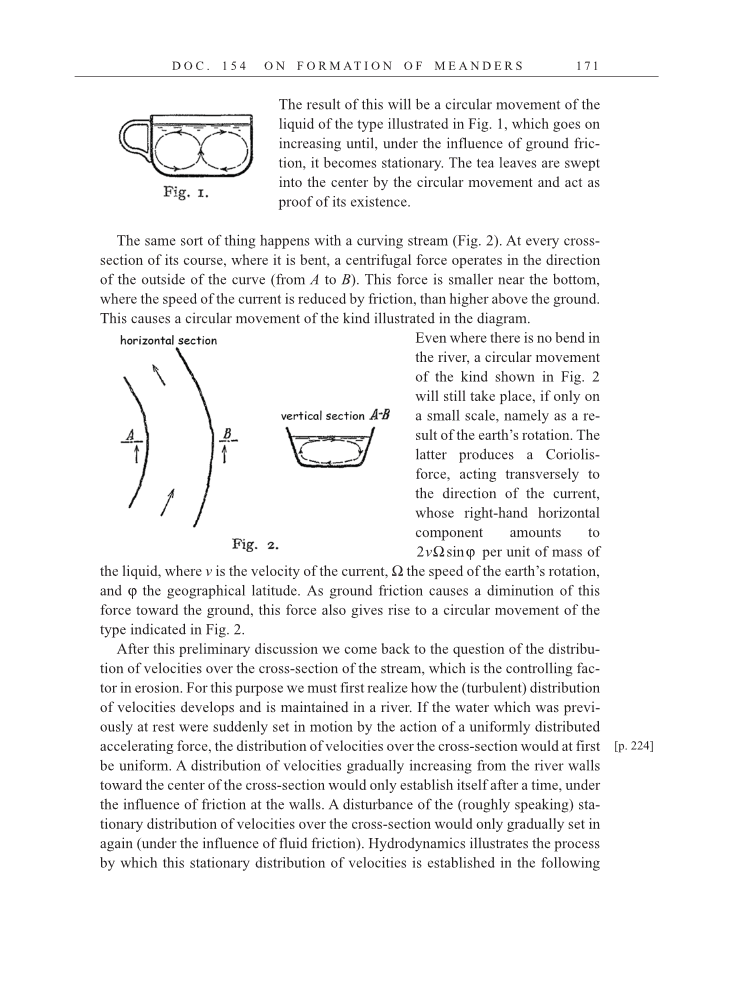
D O C . 1 5 4 O N F O R M AT I O N O F M E A N D E R S 1 7 1 The result of this will be a circular movement of the liquid of the type illustrated in Fig. 1, which goes on increasing until, under the influence of ground fric- tion, it becomes stationary. The tea leaves are swept into the center by the circular movement and act as proof of its existence. The same sort of thing happens with a curving stream (Fig. 2). At every cross- section of its course, where it is bent, a centrifugal force operates in the direction of the outside of the curve (from A to B). This force is smaller near the bottom, where the speed of the current is reduced by friction, than higher above the ground. This causes a circular movement of the kind illustrated in the diagram. Even where there is no bend in the river, a circular movement of the kind shown in Fig. 2 will still take place, if only on a small scale, namely as a re- sult of the earth’s rotation. The latter produces a Coriolis- force, acting transversely to the direction of the current, whose right-hand horizontal component amounts to per unit of mass of the liquid, where v is the velocity of the current, Ω the speed of the earth’s rotation, and ϕ the geographical latitude. As ground friction causes a diminution of this force toward the ground, this force also gives rise to a circular movement of the type indicated in Fig. 2. After this preliminary discussion we come back to the question of the distribu- tion of velocities over the cross-section of the stream, which is the controlling fac- tor in erosion. For this purpose we must first realize how the (turbulent) distribution of velocities develops and is maintained in a river. If the water which was previ- ously at rest were suddenly set in motion by the action of a uniformly distributed accelerating force, the distribution of velocities over the cross-section would at first be uniform. A distribution of velocities gradually increasing from the river walls toward the center of the cross-section would only establish itself after a time, under the influence of friction at the walls. A disturbance of the (roughly speaking) sta- tionary distribution of velocities over the cross-section would only gradually set in again (under the influence of fluid friction). Hydrodynamics illustrates the process by which this stationary distribution of velocities is established in the following 2vΩ sinϕ [p. 224]