140
DOC.
11
ARGUMENTS FOR MOLECULAR
AGITATION
Cr
Fig. 2.
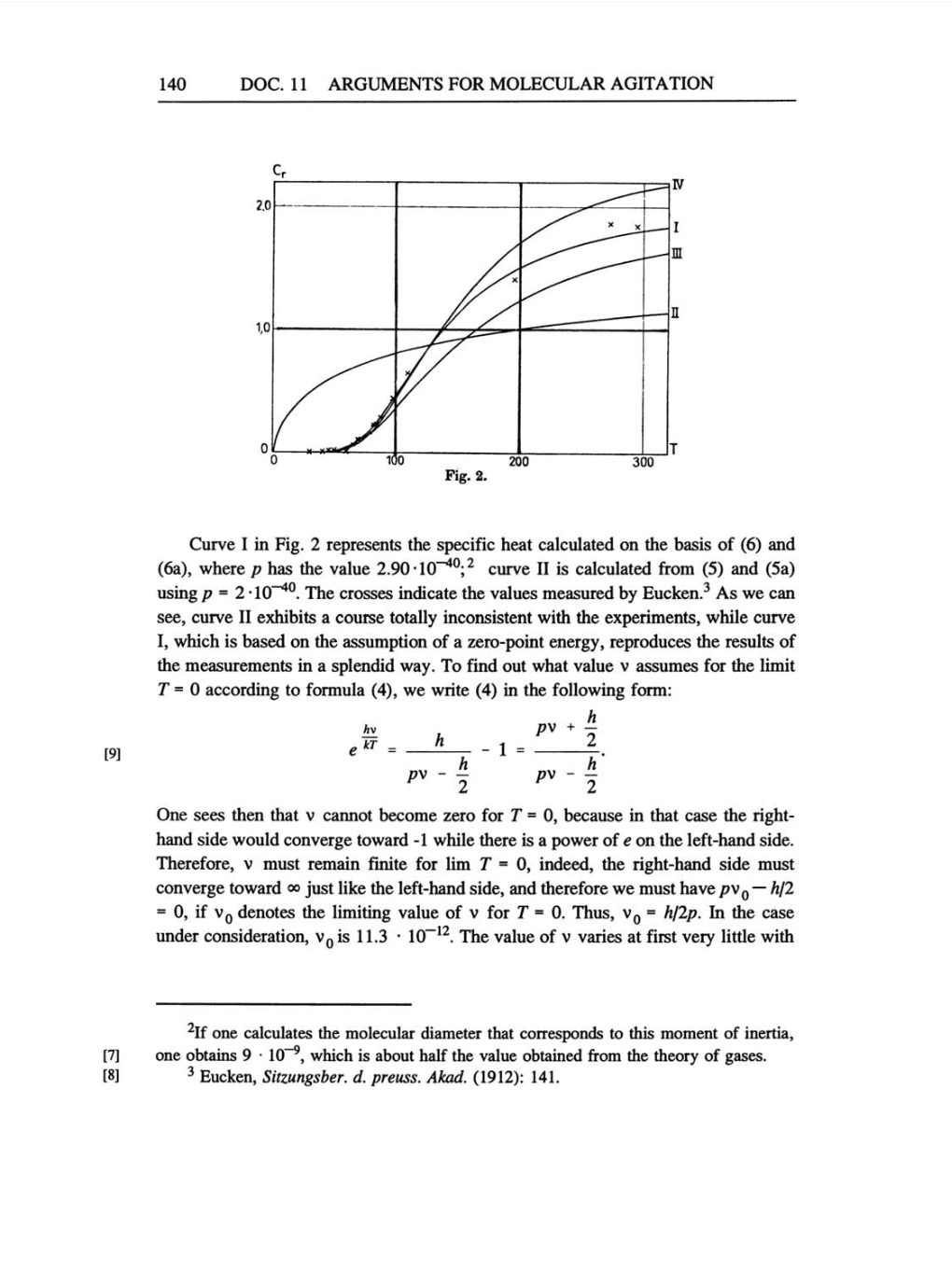
Curve
I
in
Fig.
2
represents
the
specific
heat calculated
on
the basis of
(6)
and
(6a),
where
p
has the
value
2.90.10-40;2
curve
II is calculated
from
(5)
and
(5a)
using p
=
2.10-40. The
crosses
indicate the values measured
by
Eucken.3 As
we can
see,
curve
II exhibits
a course
totally
inconsistent with the
experiments,
while
curve
I,
which is based
on
the
assumption
of
a
zero-point energy, reproduces
the results
of
the
measurements
in
a
splendid way.
To find
out
what value
v
assumes
for the limit
T
=
0
according
to
formula
(4), we
write
(4)
in the
following
form:
[9]
h
hi
,
pv
+
-
e
xt
=
h_
_
1
=2
p\
-
h.
py,
-h
2
2 2
One
sees
then that
v
cannot
become
zero
for
T
=
0,
because in that
case
the
right–
hand side would
converge
toward
-1
while there
is
a
power
of
e on
the left-hand side.
Therefore,
v
must
remain
finite for lim
T
=
0,
indeed,
the
right-hand
side
must
converge
toward
oo
just
like the
left-hand
side,
and therefore
we
must
have
pv0
-
h/2
=
0,
if
v0
denotes the
limiting
value of
v
for
T
=
0.
Thus,
v0
=
h/2p.
In the
case
under
consideration,
v0
is 11.3
.
10-12.
The value of
v
varies
at
first
very
little with
2If
one
calculates the molecular diameter that
corresponds
to
this
moment
of
inertia,
one
obtains 9
.
10-9,
which is about
half
the value obtained from the
theory
of
gases.
3
Eucken, Sitzungsber.
d.
preuss.
Akad.
(1912):
141.
[7]
[8]